Odds Of Royal Flush Holdem
- Odds Of Flopping A Royal Flush In Hold'em
- Odds Of Royal Flush Hold Em
- Odds Of Royal Flush Texas Holdem
- Odds Of Hitting A Royal Flush In Hold'em
- Odds Of Getting Royal Flush In Holdem

Odds Of Royal Flush In Holdem. To £400 in bonuses + 100 bonus spins. 18+ New eligible UK players only. Select Casino offer on sign-up and deposit. 4 deposits of £10, £20, £50, £100 matched with a bonus cash offer of same value (14 day expiry). 35x real money cash wagering (within 30 days) on eligible games Odds Of Royal Flush In Holdembefore bonus cash is credited. There are 2598960 unique 5-card poker hands (C(n,r) = C(52, 5) = 2598960). 4 of those are royal flushes. So, the odds of one specific player flopping a royal flush would be 4-in-2598960, or 1-in-649740.
- Whereas a pair floats by often enough, getting a straight or royal flush is less likely. 7 out of 52 means, that although you build your hand using 5 cards, you still have 7 cards from which to choose these 5. In the case of Texas Hold'em, there's the 2 pocket cards and 5 on the board.
- In Texas Hold'em, there are a total of 2,598,960 different five card poker hands. This includes the four royal flushes (Diamonds, Spades, Clubs and Hearts). So - the odds of hitting a royal flush would be 4/2,598,960, which would work out to 1/649,740. So, you should hit a royal flush every 650,000 hands that you play or so.
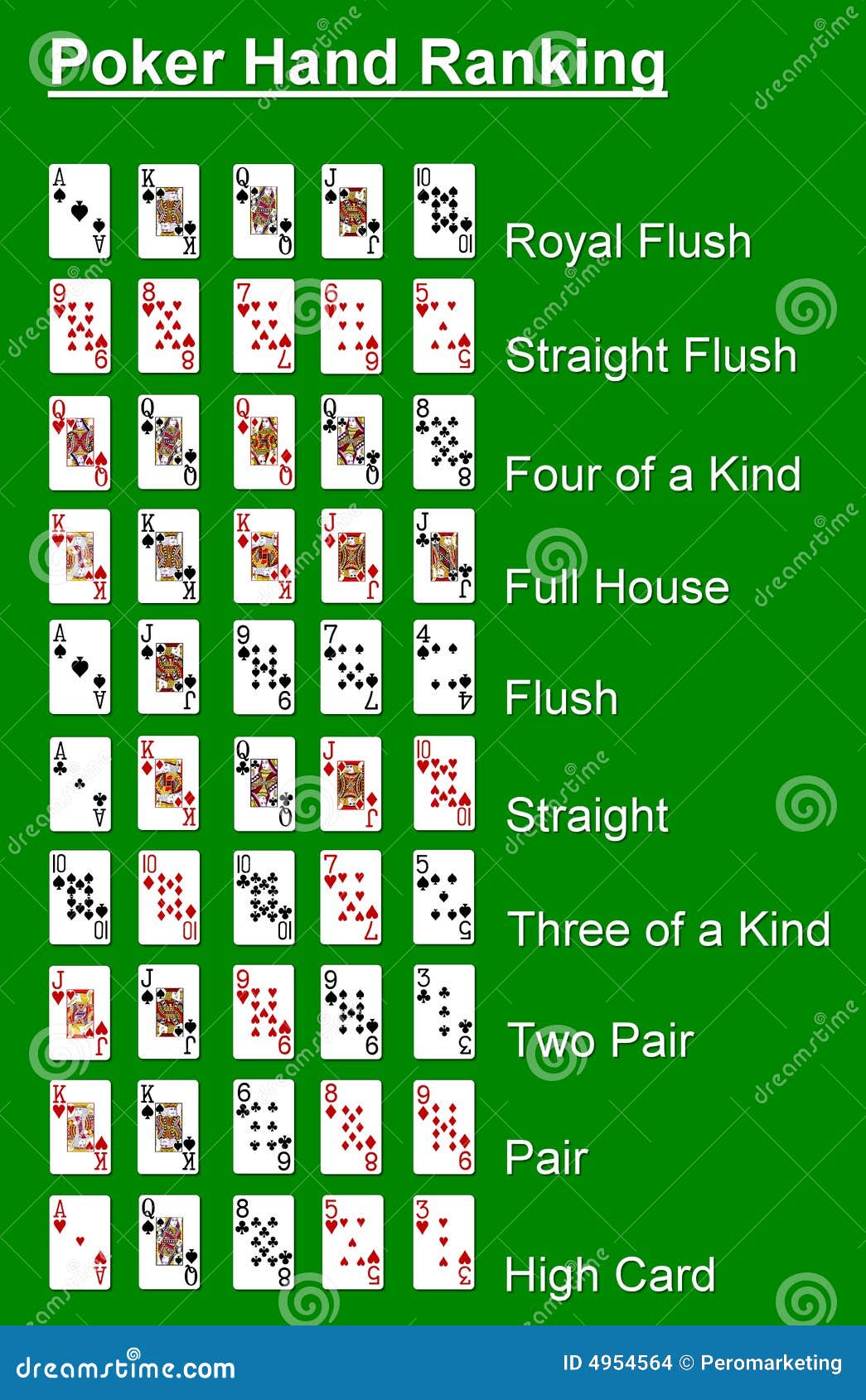
- The king of all poker hands is the royal flush. With a royal flush, it’s essentially a very specific straight flush. For starters, all your five cards must be the same suit. On top of that, it must be the 10, J, Q, K, and A of a particular suit to complete the royal flush. Poker Hand Odds for Five-Card Games.
You have 2 of the 5 RF cards in the hole. Doesn't matter if they are As-Ks or Js-Ts, etc. What are the odds you will get a Royal Flush by street:
a) make RF by the Flop
b) make RF by the Turn
c) make RF by the River
My calculations were as follows:
a1) 19,599 to 1 on the flop
b1) about 5,000 to 1 on the turn
c1) about 2,000 to 1 on the river
The general consensus was the true value by the river was either 60,000 to 1 or 30,000 to 1 by the river. This seems totally nonsensical to me but I was in the distinct minority (i.e. it was only me!). Can someone with more probability know-how step up and provide a definite answer to this question?
Thank You.
Odds Of Flopping A Royal Flush In Hold'em
My local casino runs a special prize for making a Royal Flush (RF) hand in Hold Em poker. The hand does not have to go to showdown, but both your hole cards must play (i.e. they must be 2 of the 5 RF cards). I was discussing the odds of making such a hand with other players and I got a lot of different feedback, none of which I felt was correct. Here is the scenario:
You have 2 of the 5 RF cards in the hole. Doesn't matter if they are As-Ks or Js-Ts, etc. What are the odds you will get a Royal Flush by street:
a) make RF by the Flop
b) make RF by the Turn
c) make RF by the River
My calculations were as follows:
a1) 19,599 to 1 on the flop
b1) about 5,000 to 1 on the turn
c1) about 2,000 to 1 on the river
The general consensus was the true value by the river was either 60,000 to 1 or 30,000 to 1 by the river. This seems totally nonsensical to me but I was in the distinct minority (i.e. it was only me!). Can someone with more probability know-how step up and provide a definite answer to this question?
Thank You.
If you have 2 royal flush cards as hole cards the odds that you will make a royal flush by the river using those hole cards are combin(47,2)/combin(50,2) or 1081/2118760 or 1 in 1960. 1/10 the time you flop it. 3/10 it will be made on the turn and 6/10 on the river.
The general consensus was the true value by the river was either 60,000 to 1 or 30,000 to 1 by the river. This seems totally nonsensical to me but I was in the distinct minority (i.e. it was only me!). Can someone with more probability know-how step up and provide a definite answer to this question?
Thank You.
Because in terms of it just generally happening they were correct. It's only about one in 2000 to happen by the river AFTER you get Royal holecards dealt to you, unfortunately 97% of starting hands aren't two Royal cards.
The probability of getting 2 Royal cards to start: 4*C(5,2)/C(52,2) = 40/1326 = 0.030166
Odds Of Royal Flush Hold Em
The probability of the board containing the other 3 Royal cards: C(47,2)/C(50,5) = 1081/2,118,760 = 0.000510204The probability of both events happening for you to win the high hand jackpot: 0.030166*0.000510204 = 0.00001539 = 1 in 64,974.